Creating a Tessellation

Now that you have explored interior angles, exterior angles, and tessellations, use your knowledge to create your own tessellation. In this module, you have explored regular polygons that have tessellated the plane. In this assignment, see if you can find two or even three polygons that together will tessellate.

Use one of the options listed below to create your tessellation. After you have finished your tessellation, name the two or three polygons that make up your tessellation. Using your knowledge of interior and exterior angles, show that the angles around a point of tessellation add up to 360°. You may not use the example below for your own.

Example:

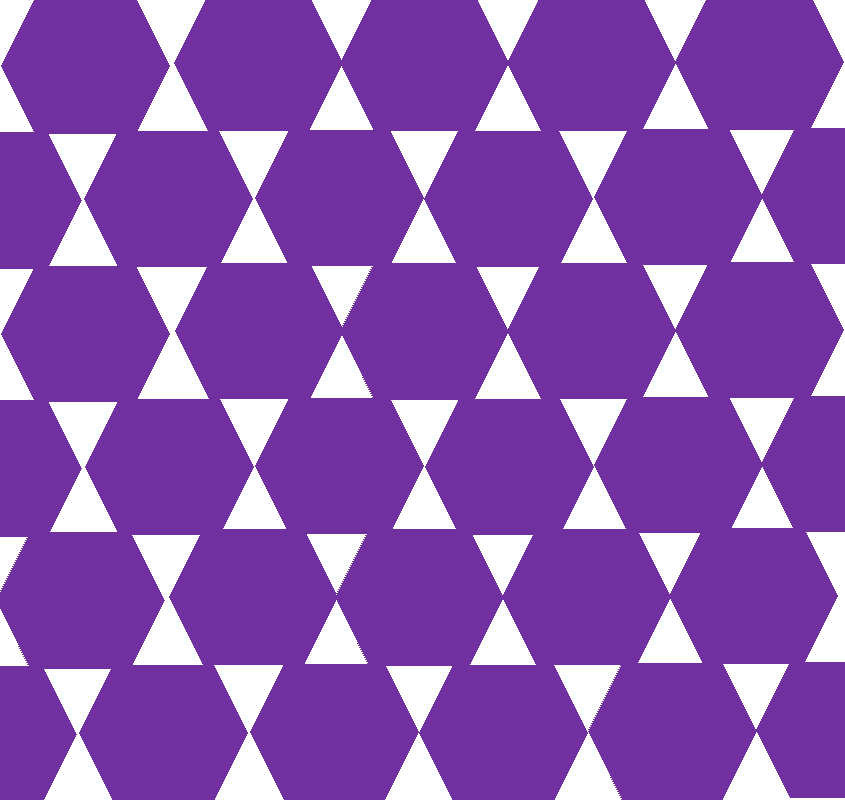
Polygons: Regular Hexagon and Regular Triangles

To find the interior angle of two regular hexagons:	To find the interior angle of two regular triangles:
First, you have to find the sum of the interior angles by using 180(n − 2). 180 (6 −2)   Substitute 6 for n. 180(4)    Simplify 720°   Multiplied Second, you need to divide the sum by the number of sides: 720/6     120°   Divided 720 by 6. Each interior angle of the regular hexagons is 120°.	First, you have to find the sum of the interior angles by using 180(n − 2). 180 (3 −2)   Substitute 3 for n. 180(1)    Simplify 180°   Multiplied Second, you need to divide the sum by the number of sides: 180/3     60°   Divided 720 by 6. Each interior angle of the regular hexagons is 60°.

Finally, add all of the interior angles: 120 + 120 + 60 + 60 = 360

Therefore, all of the interior angles around a point of tessellation add up to 360°.

Before you begin, review the Creating a Tessellation Checklist to make sure that you include all of the items required for full credit.

This activity is also available in a printable document.

 

After completing this assignment, please submit your work to the dropbox.